Building a Concave Pentakis Dodecahedron
A what now? Well, consider the humble dodecahedron. It’s got twelve pentagonal faces. Now, imagine each of those pentagons was replaced with a 5-sided pyramid. The resulting 60-face solid is called a pentakis dodecahedron. If, instead of having the pyramids on each face pointing out from the center of the solid, you have them pointing in, you end up with a different 60-face solid, the concave pentakis dodecahedron.
Of course, upon learning of such a beast, one naturally wants to make one. I started with 60 isosceles triangles. To make things interesting, I wanted a nice pattern on them. This file has half the triangles you’ll need. I used this file twice, to cut the triangles out of chipboard using Crash Space’s Epilog laser cutter.
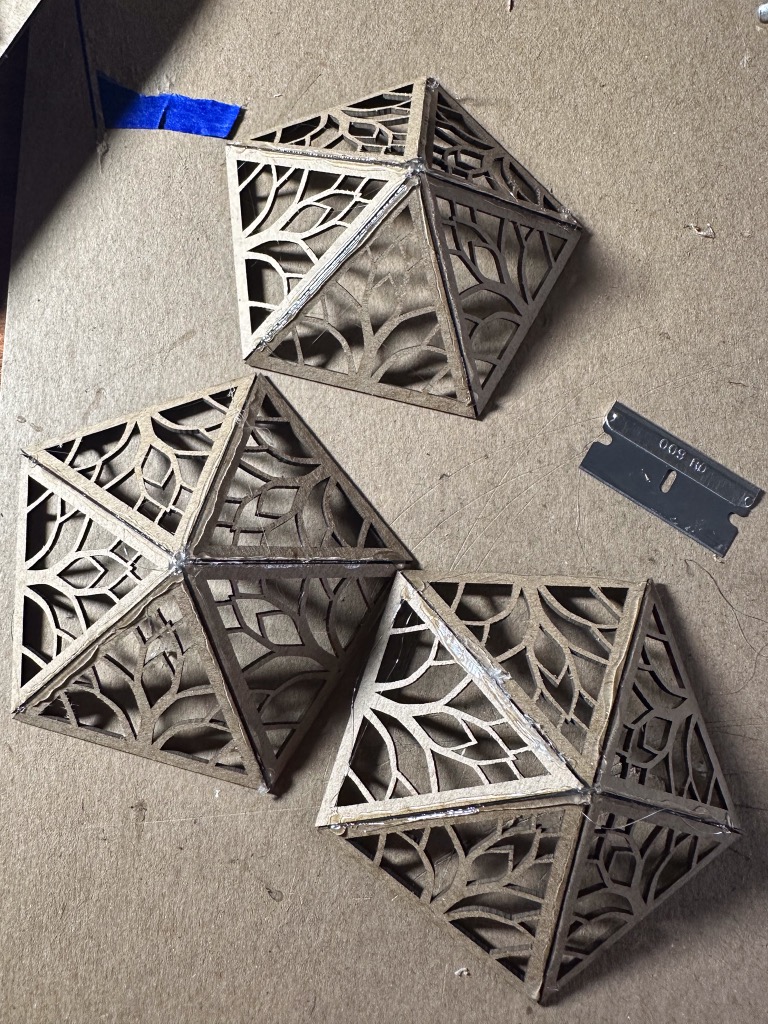
Once you have the triangles cut, there’s a tricky process of hot-gluing them together. It’s not easy holding five bits together with one hand in a very precise position, while gluing with the other. I ended up forming a jig from some discarded triangles where the pattern hadn’t cut through. The jig allowed me to place the triangles into a form and glue the point of the pyramid. With this point held in place, it was easier to complete the gluing of the rest of the pentagonal pyramid.



After a few false starts, I got the hang of it. I created the twelve pentagonal pyramids, then glued them together in sets of three. At this point, the task called for hot-glue thread cleanup. Then there was the delicate and finicky process of gluing together the four shapes to form the final “solid.”
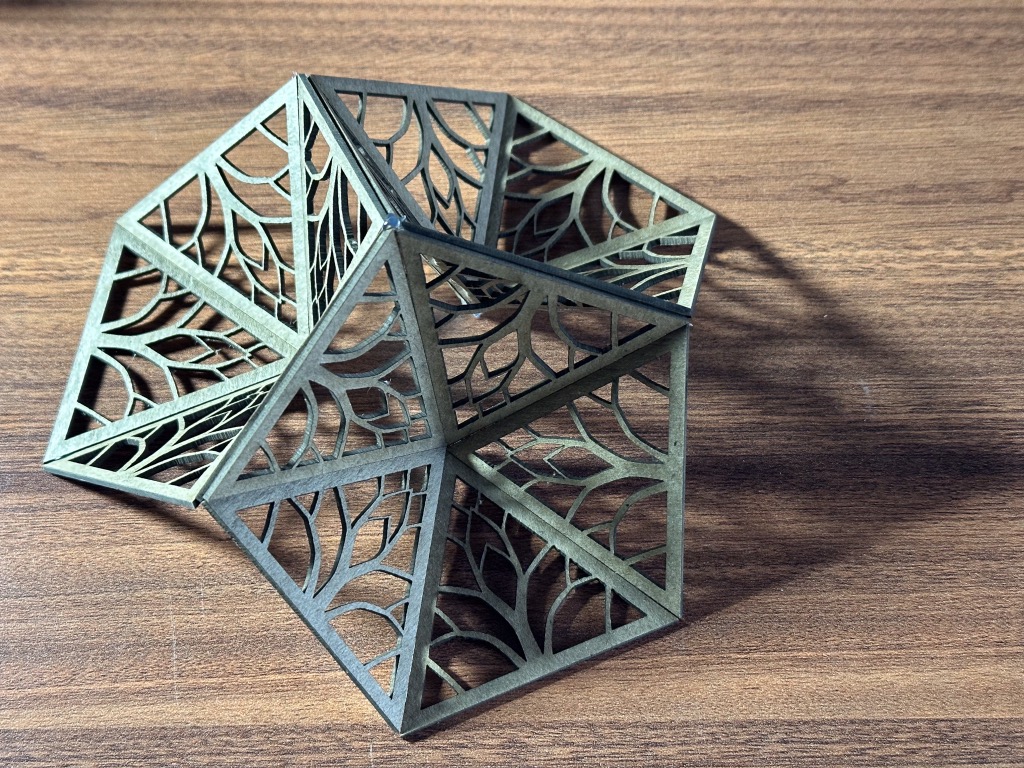
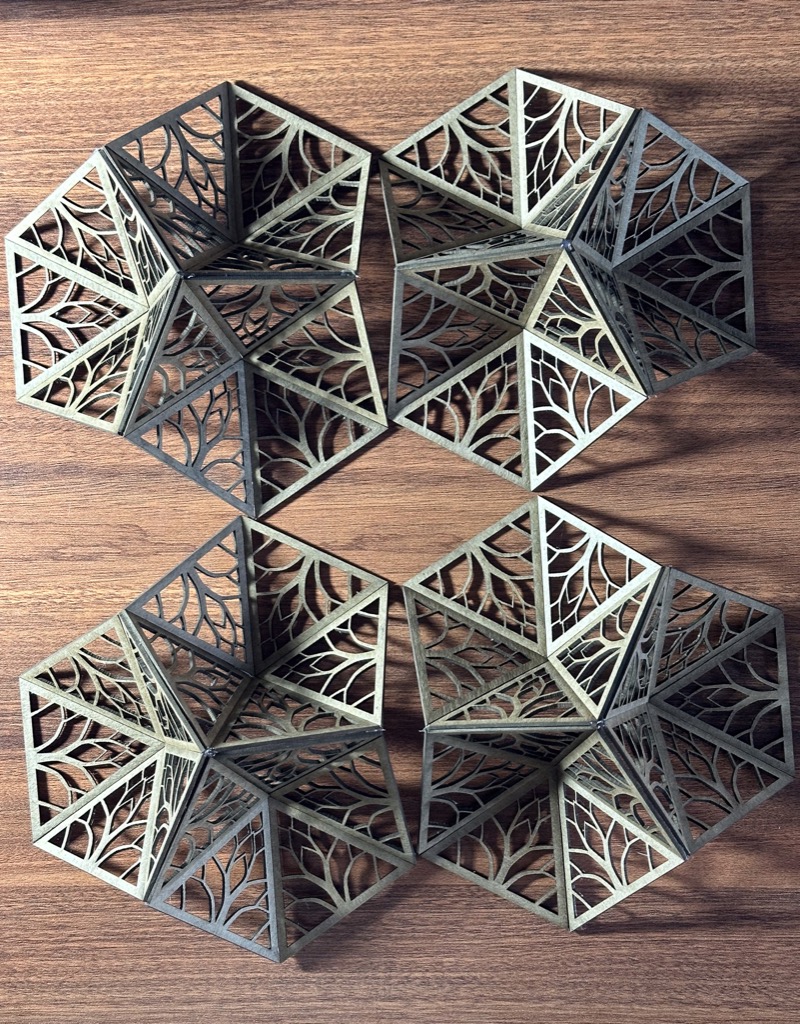

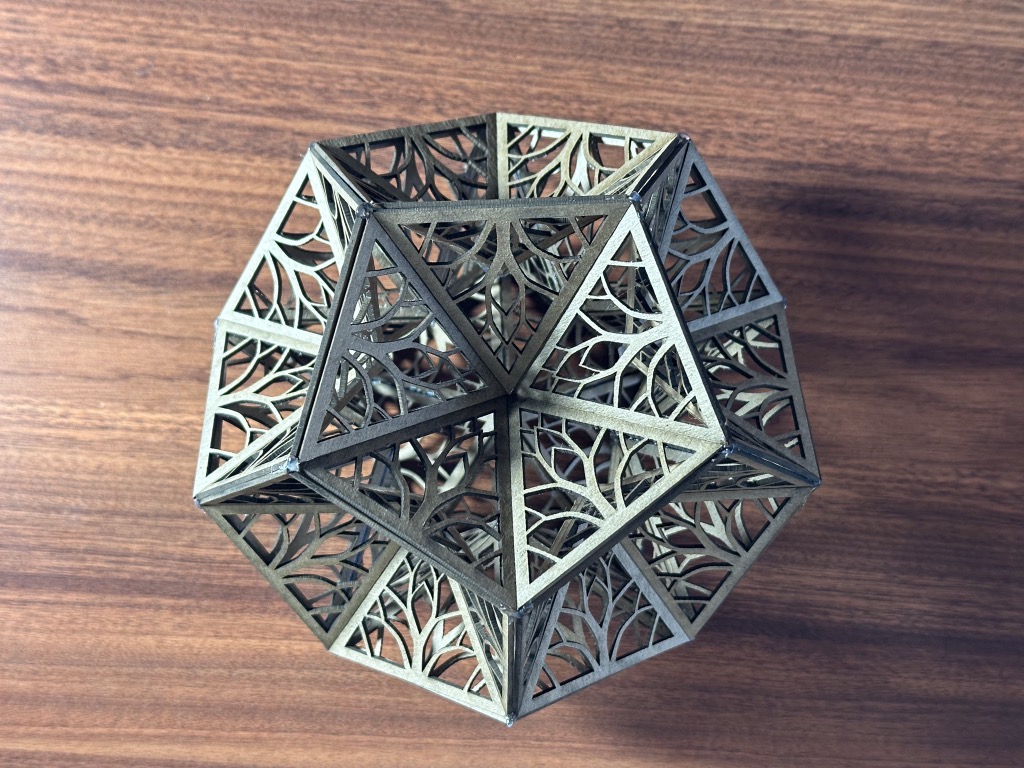
It turns out that even very small errors when gluing the initial triangles result in big errors when putting the final shape together. As I worked on it, I though the inaccuracies were appalling. But once it was complete and I viewed it from a slight distance, it wasn’t so hard to overlook the gaps and flaws.

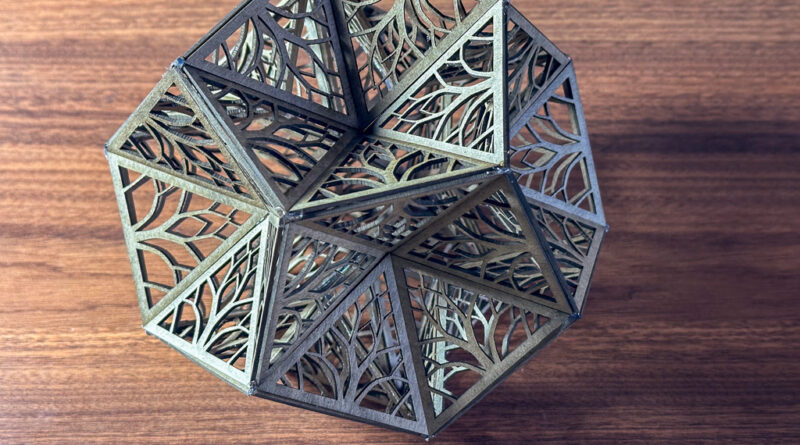
This is rad. I’ve always loved dodecahedrons since I saw MC Escher’s “Tetrahedral Planetoid” in high school, and got hooked on these beautiful shapes. Well done, indeed!